USAF-1951 test charts (also called USAF-1951 test targets) get their name from the designers and the design year: “United States Air Force 1951”. The targets are available in various finishes , for example chrome on glass.
Here is an example of how they look enlarged (source: Wikipedia)

The targets consist of “groups” of 6 “elements” each. The group numbers at the top of the group, the element numbers are located at the sides of the groups.
Each element consists of three horizontal and three vertical bars.
The camera is said to “resolve” a chart element, if the horizontal and the vertical bars can still be recognized as three distinc bars und don’t blur into one another.
When we enlarge the center of the above chart, we get: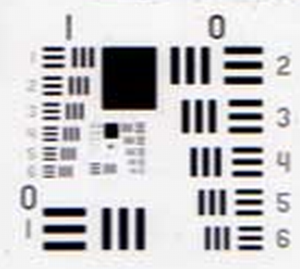
How do you interpret this? Well, we need further information, namely the width of the bars.
As expected, the width of the bars is well defined: the bar of group “group” element “element” have the width
![]()
That’s the so-called object side resolution, as obviously details of this size on the object still can be recognized.
What is the corresponding image side resolution?
If the total width of the sensor is M times larger than the total visible width of the object area (= Field Of View = FOV), then the image side resolution is by that factor M smaller than the object side resoluton!
Example:
- If we can see a total of, say, 19,2cm = 192mm of the object horizontallywith a 1/2″ sensor of 6,4mm width, the magnification M is M = 6,4 / 192 = 0,0333.
- So the image side resolution is:
image_side_resolution = 0,0333 * object_side_resolution
which in our case is approximately 157,49um * 0,0333 = 5,244um.
Example:
- If our sensor has 10um large pixels, we have found an error in our calculation! After all we can’t resolve things smaller than a pixel.
- If our sensor has 6um pixels, the resolution is approximately the size of the pixels. This is the optimum and the lens resolution is as least as good as the sensor’s.
- If our sensor has 3um large pixels, and the smallest image side resolvable details are about 5,244um, (just below 2 pixels), it could be that the lens is limiting the resolution. Or it might not be perfectly focused.
At first glance it might be a bit surprising that neither the focal length nor distance is part of the formula. But they are in fact included in the calculation, as they influence the magnification. For example the doubled distance corresponds to half the magnification.
To avoid having to use the formula again and again, here is a table (resolution in micrometer):
Resolution of the USAF1951 Target in um
| -2 | -1 | 0 | 1 | 2 | 3 | 4 | 5 | 6 | 7 | |
|---|---|---|---|---|---|---|---|---|---|---|
| 1 | 2000,0 | 1000,0 | 500,0 | 250,0 | 125,0 | 62,5 | 31,3 | 15,6 | 7,8 | 3,9 |
| 2 | 1781,8 | 890,9 | 445,4 | 222,7 | 111,4 | 55,7 | 27,8 | 13,9 | 7,0 | 3,5 |
| 3 | 1587,4 | 793,7 | 396,9 | 198,4 | 99,2 | 49,6 | 24,8 | 12,4 | 6,2 | 3,1 |
| 4 | 1414,2 | 707,1 | 353,6 | 176,8 | 88,4 | 44,2 | 22,1 | 11,0 | 5,5 | 2,8 |
| 5 | 1259,9 | 630,0 | 315,0 | 157,5 | 78,7 | 39,4 | 19,7 | 9,8 | 4,9 | 2,5 |
| 6 | 1122,5 | 561,2 | 280,6 | 140,3 | 70,2 | 35,1 | 17,5 | 8,8 | 4,4 | 2,2 |