is a mapping invariant of paraxial optics, given by the product
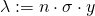
where n is the refraction index, 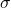 is the aperture angle, and y is the object height.
is the aperture angle, and y is the object height.
This value doesn’t change, if the object side values are replaced by the corresponding image side values:
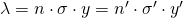
From this we get the paraxial Magnification:
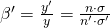
When a
lens is focussed to the hyperfocal distance H, the
DOF of the
lens is
maximized: The range of acceptable sharpness then extends from
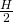
to infinity
There are two Formulas in use:
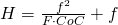
For f=50mm, F2, and CoC = 0.03mm we get

This is the formula we use here. The results just differ in the focal length of the lens.
and
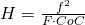
For f=50mm, F2, and CoC = 0.03mm we get
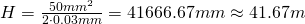
Where CoC is the circle of confusion, F is the F-number and f is the focal length of the lens.
The hyperfocal distance has curious mathematical properties:
The hyperfocal distance H is the distance at which you have to focus an object to receive the largest depth of field. If, namely, a lens is focussed to H, it is focused from 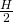 to infinity.
to infinity.
When focussed to
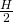
, so everything from

to
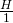
focused.
When focussed to

, so everything from
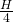
to
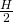
focused.
When focussed to
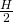
, so everything from
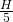
to

focused.
…
When focussed to
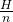
, so everything from
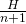
to
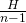
focused.
The distance
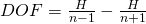
is the Depth of field.
Notice:
![]()
![]() is the aperture angle, and y is the object height.
is the aperture angle, and y is the object height.![]()
![]()