Archives

2 A B C D E F G H I K L M N O P R S T U V W
De Di Do Dw

Depth of Field

range in working distance for which the image is (acceptably) focused.

Image: Depth Of Field

Depth Of Field

DOF =  Far Point – Near Point

The largest depth of field (namely infinity) we get when we focus the lens to the so called hyperfocal distance. The focus extends from H/2 to infinity.

see also Bokeh


dispersion

(from latin dispergere, “to scatter”, to disperse” ) :

Dependency of a measure on frequency / wavelength.

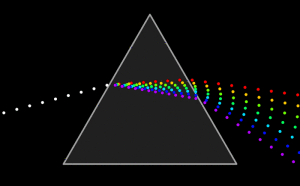Light_dispersion_conceptual(C) Wikipedia, zum Animieren bitte klicken

Using a Prism dispersion leads to splitting of white light beam into individual colors. A rainbow where light takes different paths inside the water dropplets, depending on their wavelength is another “real world” example of dispersion.

Every optical medium / glass type has different refraction indices for the various wavelength of light. The number that describes how different the light paths of the various wavelengths are, is the Abbe-number.

UNder dispersion formulas you find the most common formulas

Dispersion Formulas

Each optical material (glasses, plastics, gases) have a different refraction index for each wavelength.

Instead of keeping long tables, it’s possible to describe the behaviour of optical materials by formulas.

here are the main formulas used :

1: Sellmeier (preferred)
n^2-1=C_1 + \frac{C_2 \lambda^2}{\lambda^2-C_3^2} + \frac{C_4 \lambda^2}{\lambda^2-C_5^2} + \frac{C_6 \lambda^2}{\lambda^2-C_7^2} + \frac{C_8 \lambda^2}{\lambda^2-C_9^2} + \frac{C_{10} \lambda^2}{\lambda^2-C_{11}^2} + \frac{C_{12} \lambda^2}{\lambda^2-C_{13}^2} + \frac{C_{14} \lambda^2}{\lambda^2-C_{15}^2} + \frac{C_{16} \lambda^2}{\lambda^2-C_{17}^2}

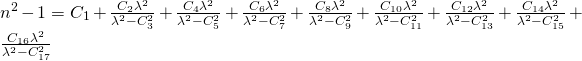
2: Sellmeier-2
n^2-1=C_1 + \frac{C_2 \lambda^2}{\lambda^2-C_3} + \frac{C_4 \lambda^2}{\lambda^2-C_5} + \frac{C_6 \lambda^2}{\lambda^2-C_7} + \frac{C_8 \lambda^2}{\lambda^2-C_9} + \frac{C_{10} \lambda^2}{\lambda^2-C_{11}} + \frac{C_{12} \lambda^2}{\lambda^2-C_{13}} + \frac{C_{14} \lambda^2}{\lambda^2-C_{15}} + \frac{C_{16} \lambda^2}{\lambda^2-C_{17}}

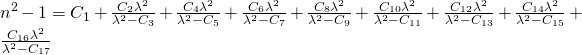
3: Polynomial
n^2 = C_1 + C_2 \lambda^{C_3} + C_4 \lambda^{C_5} + C_6 \lambda^{C_7} + C_8 \lambda^{C_9} + C_{10} \lambda^{C_{11}} + C_{12} \lambda^{C_{13}} + C_{14} \lambda^{C_{15}} + C_{16} \lambda^{C_{17}}

4: RefractiveIndex.info
n^2 = C_1 + \frac{C_2 \lambda^{C_3}}{\lambda^2-{C_4}^{C_5}} + \frac{C_6 \lambda^{C_7}}{\lambda^2-{C_8}^{C_9}} + C_{10} \lambda^{C_{11}} + C_{12} \lambda^{C_{13}} + C_{14} \lambda^{C_{15}} + C_{16} \lambda^{C_{17}}

5: Cauchy
n = C_1 + C_2 \lambda^{C_3} + C_4 \lambda^{C_5} + C_6 \lambda^{C_7} + C_8 \lambda^{C_9} + C_{10} \lambda^{C_{11}}

6: Gases
n-1 = C_1 + \frac{C_2}{C_3-\lambda^{-2}} + \frac{C_4}{C_5-\lambda^{-2}} + \frac{C_6}{C_7-\lambda^{-2}} + \frac{C_8}{C_9-\lambda^{-2}} + \frac{C_{10}}{C_{11}-\lambda^{-2}}

7: Herzberger
n = C_1 + \frac{C_2}{\lambda^2-0.028} + C_3 (\frac{1}{\lambda^2-0.028})^2 + C_4 \lambda^2 + C_5 \lambda^4 + C_6 \lambda^6

8: Retro
\frac{n^2-1}{n^2+2} = C_1 + \frac{C_2 \lambda^2}{\lambda^2-C_3} + C_4 \lambda^2

9: Exotic
n^2 = C_1 + \frac{C_2}{\lambda^2-C_3} + \frac{C_4 (\lambda-C_5)}{(\lambda-C_5)^2 + C_6}