Most distant point on the optical axis with an image of “acceptable sharpness”
![]()
Where CoC is the Circle of Confusion (the largest accepted Airy-disk) in Millimeter.
Alternatively, we can express the FarPoint using the magnification M :
![]()
If we use for example a 1/2.5″ 5 Aptina Megapixel greyscale Sensor mit 2.2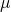 pixel pitch, we can use the pixel diagonal as CoC for crisp images, say
pixel pitch, we can use the pixel diagonal as CoC for crisp images, say 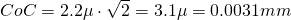
A 5 Mega lens with f=7.2mm focal length and F-stop F2.4, focused to an object distance of 100mm then has a far point of
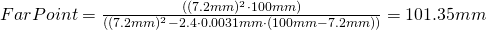
und einen Nahpunkt von
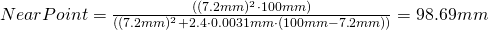
and thus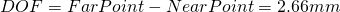
A 5 Mega lens with f=7.2mm focal length and F-stop F2.4, focused to an object distance of 100mm then has a far point of
und einen Nahpunkt von
and thus
If instead we use a 5 Megapixel greyscale Sony Sensor with 3.45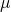 pixel pitch, we can choose as CoC the diagonal of the pixel for crisp images, say
pixel pitch, we can choose as CoC the diagonal of the pixel for crisp images, say 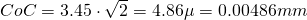
A 5 Mega lens with f=7.2mm focal length and F-stop F2.4, focussed to 100mm then results in
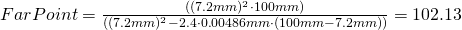 mm
mm
und einen Nahpunkt von
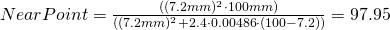
thus we get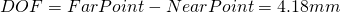
A 5 Mega lens with f=7.2mm focal length and F-stop F2.4, focussed to 100mm then results in
und einen Nahpunkt von
thus we get
If we use a color sensor instead we can use 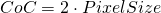 for crisp images. For the two sensors above we then get:
for crisp images. For the two sensors above we then get:
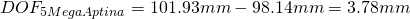
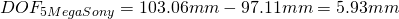
To increase the DOF we can increase the Pixel Size, but we either lose resolution, or (at the same pixel count) the magnification changes)
If you change the focal length of a lens in a way, that (with the same sensor) you get the same FOV (then from a different distance) this results in the same DOF !!!
see also https://www.optowiki.info/blog/can-i-increase-the-dof-by-changing-the-focal-length/
see also https://www.optowiki.info/blog/can-i-increase-the-dof-by-changing-the-focal-length/
When a lens is focussed to the hyperfocal distance H, the far point is at 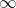 and the near point is at
and the near point is at 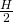 .
.
The DOF is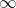 then, thus focussing to the hyperfocal distance results in the largest possible DOF.
then, thus focussing to the hyperfocal distance results in the largest possible DOF.
The DOF is